
In Physics, frequency in units of Hz is defined as the number of cycles per unit time. Angular frequency is the rate of change of phase of a sinusoidal waveform with units of radians/second.
\begin
2\pi f = \frac
\end
where $\Delta\theta$ and $\Delta t$ are the changes in phase and time, respectively. A Carrier Frequency Offset (CFO) usually arises due to two reasons. The video below also explains this concept.
To see the effect of the carrier frequency offset $F_\Delta$, consider again a received passband signal consisting of two PAM waveforms in $I$ and $Q$ arms.
\begin
r(t) &= v_I(t) \sqrt \cos \Big[2\pi (F_C+F_\Delta) t + \theta_\Delta\Big] – v_Q(t) \sqrt\sin \Big[ 2\pi (F_C+F_\Delta) t + \theta_\Delta \Big]\nonumber\\
&= v_I(t) \sqrt \cos \Big[2\pi F_Ct + 2\pi F_\Delta t + \theta_\Delta\Big] – \nonumber \\ &\hspacev_Q(t) \sqrt\sin \Big[ 2\pi F_C t+ 2\pi F_\Delta t + \theta_\Delta\Big]\label
\end
Here, the impact of carrier offset can be seen as $2\pi F_\Delta t$ which is changing the phase with time. Let us look into how to find $F_>$, the maximum value CFO can take.
The accuracy of local oscillators in communication receivers is defined in terms of ppm (parts per million) . 1 ppm is just what it says: 1 out of $10^6$ parts. To get a feel of how big or small this number is, $10^6$ seconds translate into
\begin
\frac> \times 3600 ~\text> \approx 11.5~ \text
\end
Hence, $1$ ppm is equivalent to a deviation of $1$ second every $11.5$ days. This might sound entirely harmless but for the purpose of typical wireless communication systems operating at several GHz of carrier frequency $F_C$ and several MHz of symbol rates $R_M$, it is one of the major sources of signal distortion.
The ppm rating at the oscillator crystal indicates how much its frequency may deviate from the nominal value. Consider an example of a wireless system operating at $2.4$ GHz and with $\pm 20$ ppm crystals, which is a more or less standard rating. The maximum deviation of the carrier frequency at the Tx or Rx can be
\begin
\pm \frac \times 2.4 \times 10^9 = \pm 48 \text
\end
However, in the worst case scenario, the Tx can be $20$ ppm above (or below) the nominal frequency, while the Rx can be $20$ ppm below (or above) the nominal frequency, resulting in the overall difference of $40$ ppm between the two. So the worst case CFO due to local oscillator mismatch in this example can be
\begin
\pm 2 \times 48 = \pm 96 \text
\end
Keep in mind that this calculation is for basic precision and the actual frequency may vary depending on environmental factors, mainly the temperature and aging. Finally, a movement anywhere in the channel (whether by Tx, Rx or some other object within that environment) adds Doppler shift which can be up to several hundreds of Hz. Although this Doppler shift is much less than the oscillator generated mismatch, it severely distorts the Rx signal due to the changes it causes in the channel.
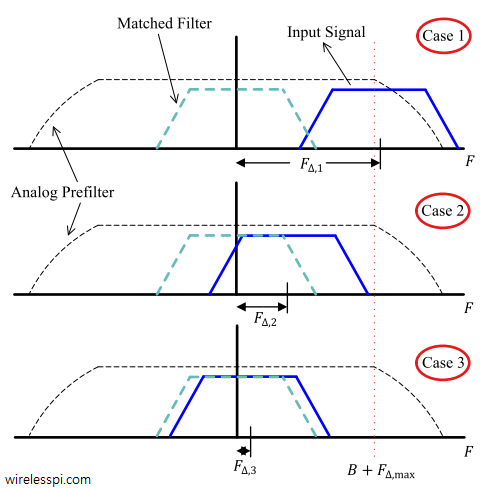
Now we turn our attention towards Figure below to differentiate between three possibilities in which $F_\Delta$ can dictate the receiver design.

It was illustrated in another article that an input signal at the Rx is filtered by an analog prefilter to remove the out of band noise. Ideally, the frequency response of this prefilter $G(F)$ should be flat within the frequency range
\begin
|F| \le B + F_>
\end
so that the incoming signal can pass through in an undistorted manner (we are assuming a flat wireless channel here as well. Actual wireless channels will be discussed later). The passband width of this prefilter is designed according to the maximum CFO $F_>$ expected at the Rx.
However, if the CFO is greater than $F_>$, then much of the actual intended signal will be filtered out by the analog prefilter and the Rx sampled signal will not even closely resemble the Tx signal as a linear function of Tx data. No amount of signal processing can then recover the signal. Since it is an outcome of poor system design, the only remedy is to redesign the system (particularly the analog frontend) with more accurate estimates and margins for CFO and other such random distortions.
Since CFO $>$, the Rx signal is within the passband of the analog prefilter $G(F)$ and suffers no distortion. However, remember from a previous post that to maximize the SNR, the Rx signal must be passed through a matched filter. This is not possible in this case because much of the Rx signal bandwidth does not sufficiently overlap with the matched filter due to the CFO being greater than $15\%$ of symbol rate $R_M$. If applied, it would remove a significant portion of the incoming signal energy.
Since Rx signal cannot be matched filtered without distorting the signal, and the signal cannot be downsampled to $1$ sample/symbol without matched filtering, it is easy to deduce that more than $1$ sample/symbol (say, $L$) is required to trace the frequency offset.
$ $15\%$ of $R_M$, an offset cycle gets completed in less than $7$ symbol times, which induces a phase rotation of more than $50^\circ$ on one symbol. Therefore, it cannot be downsampled to $1$ sample/symbol for frequency tracking. -->
When the signal is rotated by less than $15\%$ $R_M$, an offset cycle gets completed in less than $7$ symbols, and hence the effect of rotation on one symbol, although still significant, can now be tracked at symbol rate, or $1$ sample/symbol . In other words, matched filtering keeps most of the signal intact and as a result, symbol boundaries can be marked first (a problem known as symbol timing synchronization which we discuss in another article) before carrier frequency is estimated at the ISI-free symbol-spaced samples.
In the absence of noise, the sampled version of this mismatch in Eq \eqref becomes $2\pi F_\Delta nT_S$. Now Eq \eqref is very similar to phase rotation equation, and hence we can use directly use that result with proper substitution. The expression for the symbol-spaced samples in the presence of CFO $F_$ can be obtained after replacing sample time index $n$ with the symbol time index $m$.
\begin
\begin
z_I(mT_M) &= a_I[m] \cos 2\pi F_\Delta mT_M – a_Q[m]\sin 2\pi F_\Delta mT_M \\
z_Q(mT_M) &= a_I[m] \sin 2\pi F_\Delta mT_M + a_Q[m]\cos 2\pi F_\Delta mT_M
\end
\end
In the above equation,
\begin
2\pi F_\Delta mT_M = 2\pi \frac m = 2\pi F_0 m
\end
where the $F_0$ is defined as the normalized Carrier Frequency Offset (nCFO) : CFO normalized by the symbol rate.
\begin
F_0 = \frac
\end
This normalization is very important as we saw in the last subsection. The resulting expression takes the form
\begin
\begin
z_I(mT_M) = a_I[m] \cos 2\pi F_0m – a_Q[m]\sin 2\pi F_0m \\
z_Q(mT_M) = a_I[m] \sin 2\pi F_0m + a_Q[m]\cos 2\pi F_0m
\end
\end
In polar form, this expression can be written as
\begin
\begin
|z(mT_M)| &= \sqrt \\
\measuredangle z(mT_M) &= \measuredangle \Big(a_Q[m],a_I[m]\Big) + 2\pi F_0 m
\end
\end
Notice from the above equation that a carrier frequency offset of $F_\Delta$ keeps the magnitude unchanged but continually rotates the desired outputs $a_I[m]$ and $a_Q[m]$ on the constellation plane. This is drawn for symbol-spaced samples in the scatter plot of Figures below for a $4$-QAM and $16$-QAM constellation.
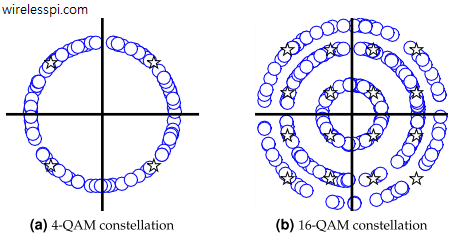
Due to this reason, a Carrier Frequency Offset (CFO), or $F_$, in the received signal spins the constellation in a circle (or multiple circles for higher-order modulations) . This is a natural outcome since the angular frequency is defined as the rate of change of phase.
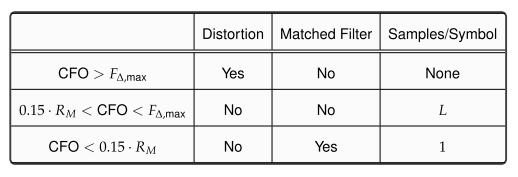
The above results are summarized in Table below.